Научный журнал

European Journal of Natural History

ISSN 2073-4972

ИФ РИНЦ = 0,301

• Авторы
• Файлы

Tanganov B.B.

Статья в формате PDF

261 KB

The article shows the possibility of applying the method of multilevel modeling in evaluating the physical and chemical variables.

In Nature, as well as in the natural sciences, everything is interconnected. This regular occurrence is usually not seen, if we bear in mind two functional parameters. But multiparameter functional relationship is observed when we apply not less than three characteristics to the sought-for parameter [1, 2, 3].

In the study of the method of multilevel modeling (MMLM), which permits to carry out mathematical modeling of certain chemical processes in different environments, and also to estimate absent (scarce) characteristics in physical-chemical methods of analysis, let´s limit with the assumption that regression is linear and is determined by the following dependence:

Y = a + b₁X₁ + b₂X₂ + ...+ b_nX_n. (1)

If we accept that a number of arguments is equal to two, then in terms of geometry, this equation defines the plane in the space of variables X₁, X₂ and Y.

To determine parameters a, b₁, ... b_n in equation (1) let´s apply the method of least squares. After differentiation according to the variable parameters this method leads to the system:

Σy = na + b₁ΣX₁ + ... + b_nΣX_n ; (2-a)

ΣyX₁ = aΣX₁ + b₁ΣX₁² + ... + b_nΣX₁X₂...X_n ; (2-b)

. . . . . . . . . . . . . . . . . .

ΣyX_n = aΣX_n + b₁ΣX₁X₂ + ... + b_nΣX_n². (2-c)

To solve this system we divide equation (2-a) to n, then we obtain:

a = y_av - b₁X_1(av) - b₂X_2(av) - ... - b_nX_n(av).

Substituting this value for а in formula (1) and equation (2-b) and (2-c), we find out that the formula MMLM with n variables has the following form:

Y-y_av = b₁(X₁-X_1(av))  + b₂(X₂ - X_2(av) ) + ... +
+ b_n(X_n - X_n(av) ), (3)

the coefficients b₁, b₂, ..., b_n are found from the following system of linear equations:

b₁Σx₁² + b₂Σx₁x₂ + ...+ b_nΣx₁x_n = Σx₁y₁ ;

b₁Σx₁x₂ + b₂Σx₂² + ...+ b_nΣx₂x_n = Σx₂y₂ ;

. . . . . . . . . . . . . . . . . .

b₁Σx₁x_n + b₂Σx₂x_n + ... + b_nΣx_n² = Σx_ny_n ,

where the following conventional signs are adopted:

Σx₁² = Σ(X₁ - X_1(av) );

Σx₁x₂ = Σ(X₁ - X_1(av) )(X₂ - X_2(av)) ;

Σx₁x_n = Σ(X₁ - X_1(av) )(X_n - X_n(av) );

Σx₁y₁ = Σ(X₁ - X_1(av) )(Y₁ - Y_1(av) ); и т.д.

Let´s point out the important physical meaning of MMLM coefficients. For example, the coefficient b₁ in equation (3) respond to the question, how many units varies Y₁, if X₁ changes by one unit on the assumption that X₂ retains a constant value.

Thus, the MMLM formula can eliminate the influence of factor X₂, bound with the factor of X₁ on Y in its pure form.

The problem of various characteristics optimizing of all sorts of systems becomes relevant in connection with the intensive development of the theory and practice of electrolyte solutions, physical and chemical methods of research, the development of modern methods of processing the experimental results. For example, parameters for the method of pair correlations in comparative calculations of physical-chemical properties of substances (e.g., concentration is in the functional dependence of analytical signal) in most cases are poorly known and considerably scatter or non-existent. That problem hinders their choice for a variety of assessment operations.

A common reliable regular occurrence, linking changes in various properties of complex compounds in a solvent, hasn´t been found so far, as well as the quantitative relationships between the major, basic, physical-chemical properties and various derivative properties of complex compounds in various media.

Derivation of multiple linkages and interdependence of properties and their changes for solvents, including water and electrolyte solutions is possible with deliberate and clear choice of benchmarks and with properly determined values of physical and chemical key properties of the studied systems [4].

Depending on whether a system (herein solvent) exchanges with the environment in matter and energy, it is thermodynamically isolated, closed or open and respectively is characterized by the microcanonical, canonical or macrocanonical Gibbs´s distributions. This basis must be sufficiently complete and contain at least four parameters: thermochemical, electrical, kinetic and structural parameters. Their usefulness follows from the correspondence of solvent molecules to statistical Gibbs´s ensembles with the main role of internal and external parameters:

ξ = Φ (а₁, а₂, ..., Т),

where ξ is an internal parameter, а₁, а₂, ...,Т is an external parameter.

1. It is known that if the system (herein solvent) is in equilibrium conditions without exchange with the macroscopic environment, or environment in matter and energy, it is thermodynamically isolated. In these conditions, its characteristics are determined by the parameters of the internal structure, i.e. by the length and coupling constant, atom´s masses, the number of electrons, etc.

2. If the system communicates with the environment only by energy, it is thermodynamically closed. The process can be described by thermochemical parameters of constant number of particles.

3. In case the system exchanges with the macroscopic environment both with energy and matter, the system is thermodynamically open, the number of particles in the system is variable. In such a situation the number of particles may change, to a first approximation, by the forces of electromagnetic origin, which determine the appropriate response of the electromagnetic characteristics of the investigated system.

4. Any movement of bodies at a certain speed in the condensed phase generates dissipative processes, mainly characterized by kinetic parameters: viscosity, diffusion, thermal conductivity or other parameters.

Theoretically modulating the processes of measurement, i.e. interaction between a system and a device, it is necessary to take into account all the situations observed above. This idea is the basis for the evaluation of dissociation constants of electrolytes in the studied solvents рК, the radii of solvent molecules R_s, the energies of intermolecular interactions in pure solvents ΔН and other physical and chemical characteristics of solvents and non-aqueous electrolyte solutions.

Table presents the data obtained from the thermodynamic constants of dissociation of hydrochloric acid, the titrant, in analytical chemistry рК_HCl. Its systematic values, such basic properties of the solvent as a boiling point Т, density ρ, viscosity h, a dipole moment of solvent molecule р, a molar mass of solvent М, an amount of bond lengths ΣL⋅10⁸ cm, the radius of the solvent molecule R_s⋅10⁸ cm and autoprotolysis constants (ionic product) of solvent pKs are absent.

The program «MMLM» leads to the equation

рК_HCl = - 0,03220·T - 5,08662·ρ + 0,52392·η + 2,71678·p - 0,05334·M +
+ 0,40049·ΣL + 3,40571·R_s - 0,07179·pK_s + 6,70942. (4)

multilevel factor (multidimentional) of modeling is K_ММLМ = 0,9741.

As it will be shown further, all the members of the right part of the equation have dimension mol/dm³. Dimension in other cases of MMLM (like identifying the dimension рК_HCl) are defined similarly.

The dimensional coefficients in MMLM, for example, in the same equation (4), can be obtained by solving the system of normal equations:

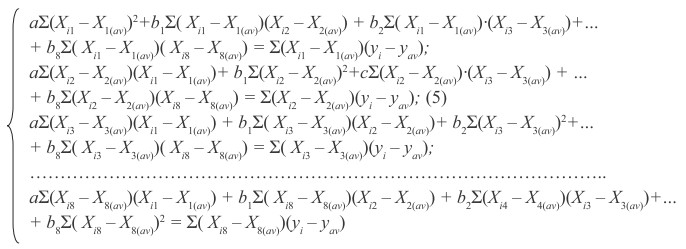
for a, b₁, b₂,... b₈, where i is a number of variables (herein the number of solvents); X_i1 = T_boiling; X_i2 is a solvent density ρ, g/сm³ ; X_i3 is viscosity of the solvent η, сПз; X_i4 is a dipole moment of solvent molecule р_i, D; Х_i5 is a molar mass of solvent М, g/mol, Х_i6 is an amount of bond lengths ΣL·10⁸ cm, Х_i7 is a radius of the solvent molecules R_s·10⁸ cm, Х_i8 is an indicator of autoprotolysis constants (ionic product) solvent pK_s, y_av, X_1(av) , X_2(av) , X_3(av) ... X_8(av) is an arithmetic functions (mathematical expectation) of the relevant parameters with the number of variables i. We obtain the following factors and their dimensions:

b₁ = - 0,03220 mol/dm³⋅К; b₂ = - 5,08662 mol/kg;

b₃ = 0,52392 mol/dm³⋅сП;

b₄ = 2,71678 mol/dm³⋅D; b₅ = -0,05334 mol²/kg,

b₆ = 0,40049 mol/dm², b₇ = 3,40571 mol/dm²,

b₈ = -0,07179 mol²/dm⁶ and а = 6,70942 mol/dm³.

The basic parameters for assessing the physical and chemical properties of solvents and the results
of evaluations of MMLM derivative characteristics

Notes: 1 - water, 2 - methanol, 3 - ethanol, 4 - propanol, 5 - butanol, 6 - pentanol, 7 - acetone, 8 - methylethylketone, 9 - metilpropilketon, 10 - metilbutilketon, 11 - dimethylformamide, 12 - dimethylacetamide, 13 - hexamethylphosphotriamide, 14 - dimethylsulfoxide, 15 - tetrametilensulfon, 16 - metilpirrolidon, 17 - acetonitrile, 18 - propylene carbonate.

Thus, when applying heterogeneous units of initial parameters Xi1, Xi2 , Xi3 , ..., Xi8 and equations of the method of multilevel modeling

y_i = y_av+ b₁(X_i1 - X_1(av) ) + b₂(X_i2 - X_2(av) ) +
+ b₃(X_i3 - X_i3(av) ) +...+ b₈(X_i8 - Х_8(av) )

units of measure and dimensional ratios yi in mol/dm3 for the thermodynamic constants of dissociation of hydrochloric acid рКHCl are obtained. The coefficient method of multilevel modeling KMMLM is introduced to assess the ties between variables. It is defined by the formula:

K_MMLM² = S(Y_i - Y_av)² / Σ(y_i - y_av)²,

where y_i is the variable Y, taken from the correlation table 1 (reference value), and Y_i is the variable Y, calculated from the equation MMLM (4).

MMLM gains an evident advantage over the method of pair correlations. It is obviously seen when comparing the coefficients of multiple regression К_MMLM and pair correlations К_pc. The ratio К_MMLM of such basic parameters as T_boiling, ρ, η, р, М, ΣL·10⁸, R_s·10⁸ and pK_s by equation (4) for water, alcohols, ketons and other solvents is equal to 0.9741, while coefficients of pair correlations рК_HCl - Т, рК_HCl - ρ, рК_HCl - η, рК_HCl - р, рК_HCl - М, рК_HCl - ΣL, рК_HCl - R_s, рК_HCl - pK_s are respectively: 0,0012; 0,0009; 0,0083; 0,1668; 0,00003; 0,15020 and 0,3713 which is considerably less than 0,9741.

Thus, using as a benchmark the thermochemical (boiling point, molar heat of vaporization, etc.) and kinetic (viscosity, etc.), electrical (dipole moment, etc.) properties and molecular characteristics (the sum of the lengths of chemical bonds in the molecule of solvent etc.) which are easily identified reference values, gives a satisfactory equivalence between the estimated values MMLM with real experimental values, regardless to the nature and class of substances. The method of multilevel (multidimensional) modeling permits to solve numerous problems in the absence of important characteristics in different branches of chemical science and technology.

Reference

1. Tanganov B.B. // Journal of physical chemistry. - 1986. - Vol. 60, № 5. - P. 1435-1438.
2. Tanganov B.B., Mognonov D.M., Nikiteev V.V., etc. // Izv. SO AN USSR. - Series of chemical sciences, 1988. - № 19, Vol. 6. - P. 105-108.
3. Tanganov B.B. // European Journal of Natupal History. - 2010. - №1. - P. 33.
4. Baldanov M.M., Tanganov B.B., Mokhosoev M.V. // Journal of physical chemistry. - 1994. - T. 65, № 2. - P. 362-367.

The work was submitted to the International Scientific Conference «New Materials and Chemical Engineering», Maldives, 16-23 March 2011. Came to the editorial office on 19.01.2011.

Библиографическая ссылка